Correlation Between the Theory of Lissajous Figures and the Generation of Pinched Hysteresis Loops in Nonlinear Circuits
In this paper, the application of the theory of Lissajous figures to the creation of pinched hysteresis loops, considered to be a characteristic of memristive systems, is demonstrated and experimentally verified using designed electronic circuits in the form of an input impedance. The relationship between the Lissajous-based model of the pinched hysteresis loop and a previously reported integrator-multiplier model is clarified. Important special cases are highlighted and necessary conditions to obtain a pinch point, loops with positive or negative inclination, as well as no pinch point are
A low frequency oscillator using a super-capacitor
A low frequency relaxation oscillator is designed using a super-capacitor. An accurate analytical expression for the oscillation frequency is derived based on a fractional-order super-capacitor model composed of a resistance in series with a Constant Phase Element (CPE) whose pseudo-capacitance and dispersion coefficient are determined using impedance spectroscopy measurements. Experimental results confirm our theoretical analysis. © 2016 Elsevier GmbH. All rights reserved.
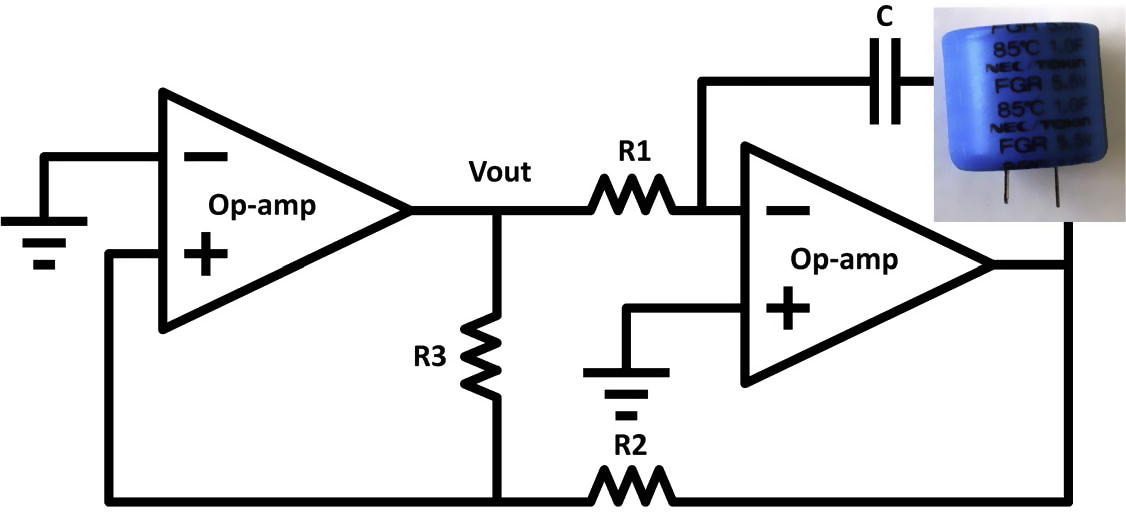
Minimal two-transistor multifunction filter design
This paper presents a comprehensive method and analysis on the design of two-transistor multi-output filters where three possible functions are simultaneously available. Although two transistors are employed at its core, proper biasing does not require additional passive components. A total of thirteen valid second-order filters are reported, and several of them are experimentally tested using discrete transistors as well as simulated using Spectre in a BiCMOS process. A fully differential realization of a MOS-C band-pass filter, based on one of the structures found, is designed and then used
On the Analysis and Design of Fractional-Order Chebyshev Complex Filter
This paper introduces the concept of fractional-order complex Chebyshev filter. A fractional variation of Chebyshev differential equations is introduced based on Caputo fractional operator. The proposed equation is solved using fractional Taylor power series method. The condition for fractional polynomial solutions is obtained and the first four polynomials scaled using an appropriate scaling factor. The fractional-order complex Chebyshev low-pass filter based on the obtained fractional polynomials is developed. Two methods for obtaining the transfer functions of the complex filter are
Software and Hardware Implementation Sensitivity of Chaotic Systems and Impact on Encryption Applications
This paper discusses the implementation sensitivity of chaotic systems added to their widely discussed sensitivities to initial conditions and parameter variation. This sensitivity can cause mismatches in some applications, which require an exact duplication of the system, e.g., chaos-based cryptography, synchronization and communication. Specifically, different implementation cases of three discretized jerk-based chaotic systems and a discrete-time logistic map are presented corresponding to different orders of additions and multiplications. The cases exhibit roughly similar attractor shapes
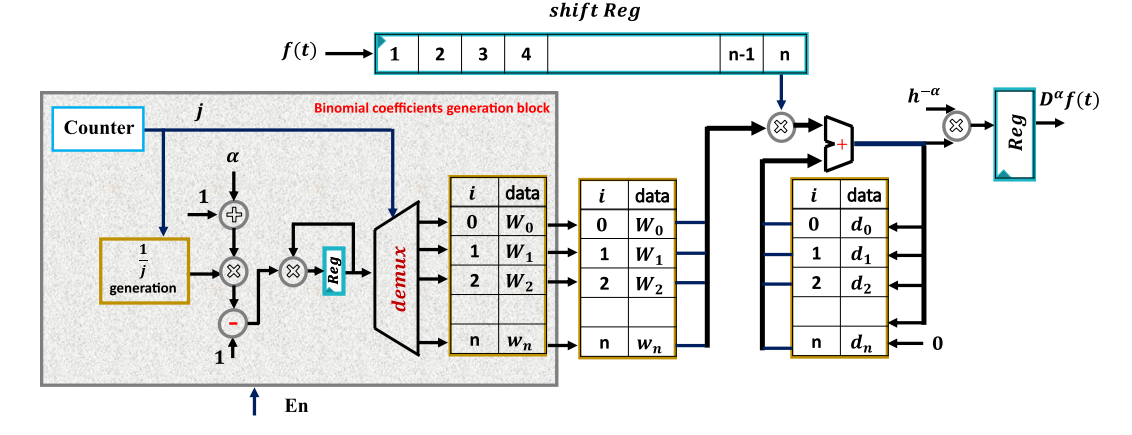
Enhanced FPGA realization of the fractional-order derivative and application to a variable-order chaotic system
The efficiency of the hardware implementations of fractional-order systems heavily relies on the efficiency of realizing the fractional-order derivative operator. In this work, a generic hardware implementation of the fractional-order derivative based on the Grünwald–Letnikov’s approximation is proposed and verified on a field-programmable gate array. The main advantage of this particular realization is its flexibility in applications which enable easy real-time configuration of the values of the fractional orders, step sizes, and/or other system parameters without changing the hardware
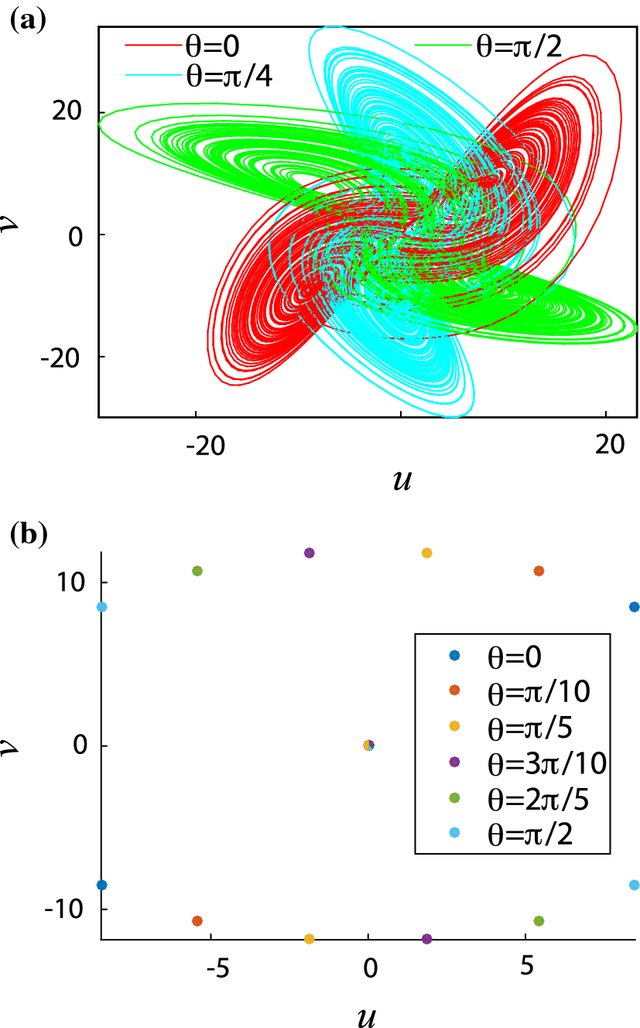
Two-Dimensional Rotation of Chaotic Attractors: Demonstrative Examples and FPGA Realization
In this work, we demonstrate the possibility of performing two-dimensional rotation on a chaotic system. This enables the rotation of its attractor in space without changing its chaotic dynamics. In particular, the rotated system preserves the same eigenvalues at all equilibrium points and its largest Lyapunov exponent remains unchanged. Two chaotic systems, one of which is the classical Lorenz system, are used to illustrate and validate the rotation operation using numerical simulations and further experimentally using a digital FPGA platform. © 2019, Springer Science+Business Media, LLC
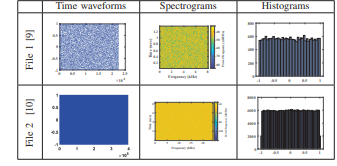
Speech Encryption on FPGA Using a Chaotic Generator and S-Box Table
In this paper, we proposed a new technique for designing a dynamic S-box depended on the idea of DNA module and Chaotic system to increase its security. Lorenz chaotic generator is utilized as the chaos part of the proposed design. This design is Tested on the Field Programmable Gate Array (FPGA) for the use of offline speech encryption and decryption in real time. The experimental results are presented on the oscilloscope. The security of the system is also validated through tests on Matlab. © 2019 IEEE.
On inverse problem of generalized synchronization between different dimensional integer-order and fractional-order chaotic systems
Chaos is described as a unstable dynamic behavior with dependence on initial conditions. The control and synchronization of chaotic systems requires the knowledge of parameters in advance. Recently researcher's has been shifted from integer order chaotic system to fraction order chaotic system. In this work, based on the stability theory of integer-order linear systems and Lyapunov stability theory, we present some control schemes to achieve a new type of synchronization called inverse generalized synchronization between different dimensional integer and fractional-orders chaotic systems. The
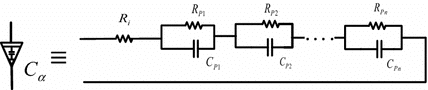
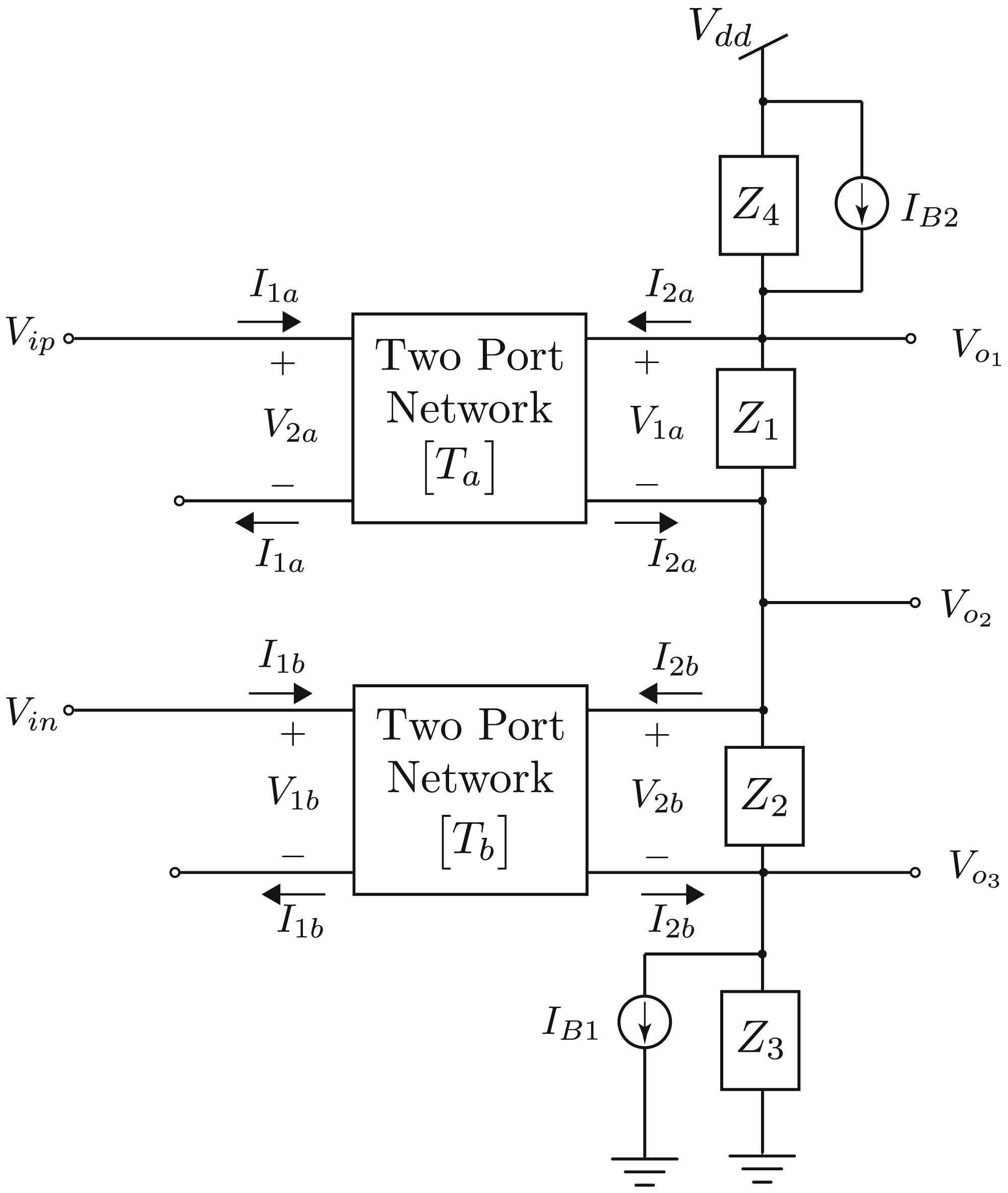
Effect of Different Approximation Techniques on Fractional-Order KHN Filter Design
Having an approximate realization of the fractance device is an essential part of fractional-order filter design and implementation. This encouraged researchers to introduce many approximation techniques of fractional-order elements. In this paper, the fractional-order KHN low-pass and high-pass filters are investigated based on four different approximation techniques: Continued Fraction Expansion, Matsuda, Oustaloup, and Valsa. Fractional-order filter fundamentals are reviewed then a comparison is made between the ideal and actual characteristic of the filter realized with each approximation
Pagination
- Previous page ‹‹
- Page 41
- Next page ››
