Towards optimum condition assessment policies for water and sewer networks
With ageing water and sewer infrastructure in North America, assessing the condition of these assets has received increased attention in the past few years. Condition assessment is an integral component in any asset management program. Determining the condition of buried infrastructure tends to be more cumbersome, costly and error-prone compared to other surface infrastructure like roads and buildings. For sewers, CCTV is considered the industry standard for condition assessment technologies. For pressurized water pipelines, technologies tend to be more costly and uncertain (e.g
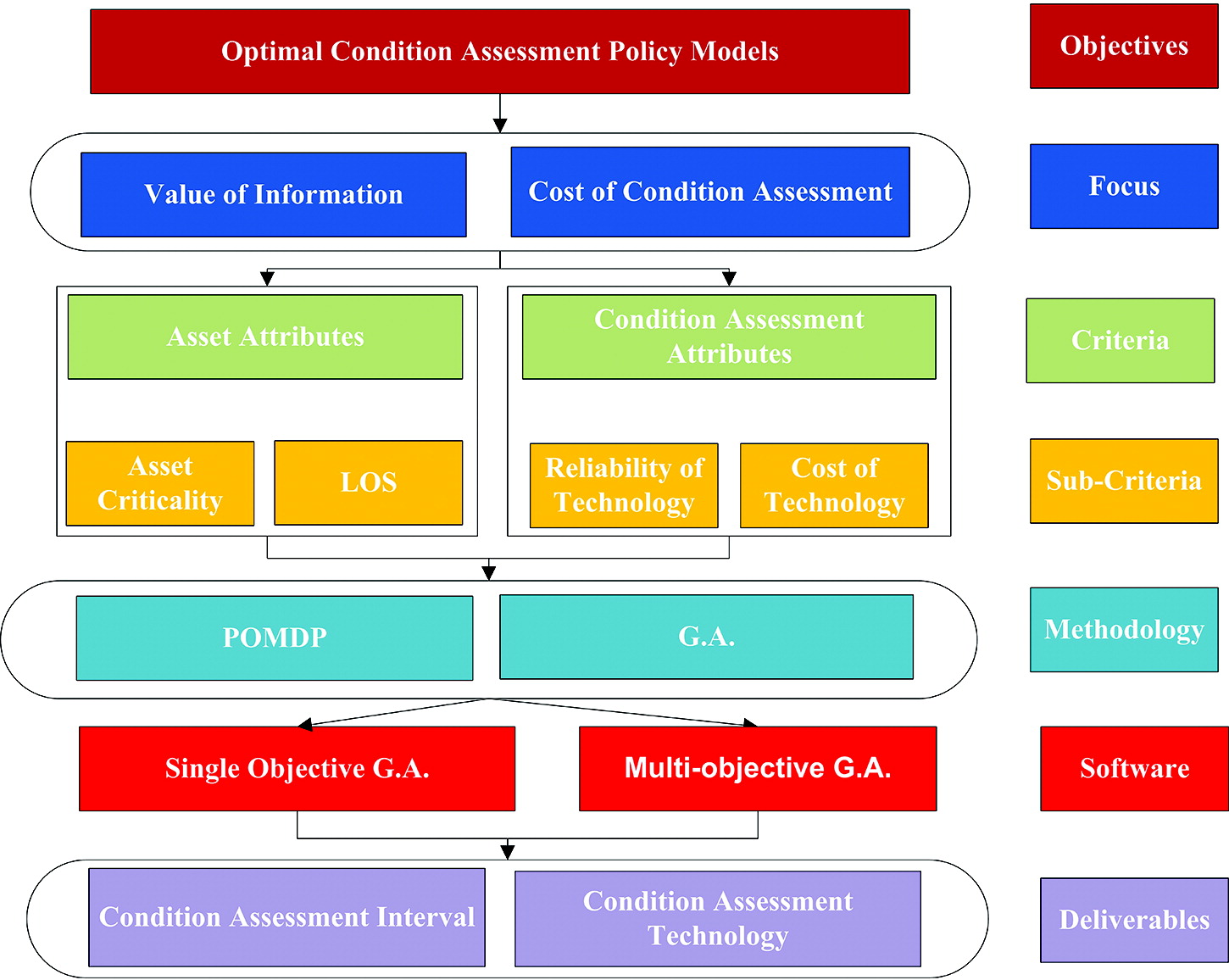
Multiobjective genetic algorithm to allocate budgetary resources for condition assessment of water and sewer networks
This paper presents a framework for optimizing condition assessment policies by balancing the revealed value of information with the cost of obtaining such information. The computational platform is based on augmenting the asset condition state with an expected level of accuracy. Inaccuracies due to condition assessment reliability are evaluated using the partially observable Markov decision process. The single objective genetic algorithm is used to select the most cost-effective assets to assess considering information inaccuracy under a fixed budget. The model is extended using
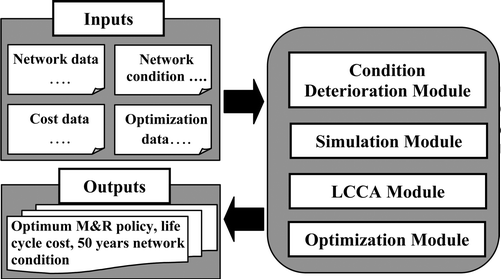
Multiobjective optimisation algorithm for sewer network rehabilitation
Understanding of deterioration mechanisms in sewers helps asset managers in developing prediction models for estimating whether or not sewer collapse is likely. Effective utilisation of deterioration prediction models along with the development and use of life cycle maintenance cost analysis contribute to reducing operation and maintenance costs in sewer systems. This article presents a model for life-cycle maintenance planning of deteriorating sewer network as a multi-objective optimisation problem that treats the sewer network condition and service life as well as life-cycle maintenance cost
Optimum Scheduling the Electric Distribution Substations with a Case Study: An Integer Gaining-Sharing Knowledge-Based Metaheuristic Algorithm
This work is dedicated to the economic scheduling of the required electric stations in the upcoming 10-year long-term plan. The calculation of the required electric stations is carried out by estimating the yearly consumption of electricity over a long-time plan and then determining the required number of stations. The aim is to minimize the total establishing and operating costs of the stations based on a mathematical programming model with nonlinear objective function and integer decision variables. The introduced model is applied for a real practical case study to conclude the number of
Analysis of Tapered Timoshenko and Euler-Bernoulli Beams on an Elastic Foundation with Moving Loads
This research studies the vibration analysis of Euler-Bernoulli and Timoshenko beams utilizing the differential quadrature method (DQM) which has wide applications in the field of basic vibration of different components, for example, pillars, plates, round and hollow shells, and tanks. The free vibration of uniform and nonuniform beams laying on elastic Pasternak foundation will be studied under three sets of boundary conditions, that is, mixing between being simply upheld and fixed while utilizing the DQM. The natural frequencies and deflection values were produced through the examination of
Two-dimensional heat conduction in a rigid thermal conductor within the dual-phase-lag model by one-sided Fourier transform
An exact analytical solution in closed form is obtained for a two-dimensional initial-boundary-value problem of heat wave propagation in a thick slab of an anisotropic rigid thermal conductor within the dual-phase-lag model. One-sided Fourier transform technique is used to obtain a formal solution. The method requires an essential change of the dependent variable in order to guarantee a suitable asymptotic time behavior of the unknown function. The solution satisfies prescribed boundary temperatures and zero initial conditions. Numerical results are presented to put in evidence the effect of
Analytic and numeric analysis for deformation of non-prismatic beams resting on elastic foundations
Background: The buckling load as well as the natural frequency under axial load for non-prismatic beam is a changeling problem. Determination of buckling load, natural frequency, and elastic deflection is very important in civil applications. The current paper used both perturbation method (PM), analytic method, and differential quadrature method (DQM), numerical method, to find buckling load and natural frequency with different end supports. The deflection of the beam resting on an elastic foundation under transverse distributed and axial loads is also obtained. Both PM and DQM are used for
Comparative Analysis of Various Machine Learning Techniques for Epileptic Seizures Detection and Prediction Using EEG Data
Epileptic seizures occur as a result of functional brain dysfunction and can affect the health of the patient. Prediction of epileptic seizures before the onset is beneficial for the prevention of seizures through medication. Electroencephalograms (EEG) signals are used to predict epileptic seizures using machine learning techniques and feature extractions. Nevertheless, the pre-processing of EEG signals for noise removal and extraction of features are two significant problems that have an adverse effect on both anticipation time and true positive prediction performance. Considering this, the
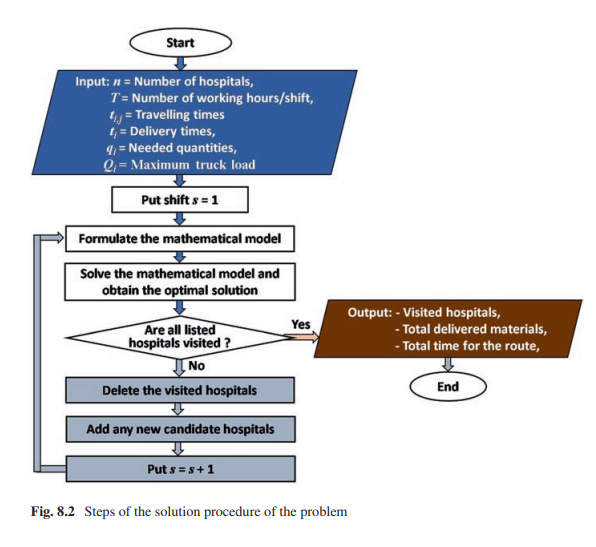
Optimum distribution of protective materials for COVID−19 with a discrete binary gaining-sharing knowledge-based optimization algorithm
Many application problems are formulated as nonlinear binary programming models which are hard to be solved using exact algorithms especially in large dimensions. One of these practical applications is to optimally distribute protective materials for the newly emerged COVID-19. It is defined for a decision-maker who wants to choose a subset of candidate hospitals comprising the maximization of the distributed quantities of protective materials to a set of chosen hospitals within a specific time shift. A nonlinear binary mathematical programming model for the problem is introduced with a real
Optimum Location of Field Hospitals for COVID-19: A Nonlinear Binary Metaheuristic Algorithm
Determining the optimum location of facilities is critical in many fields, particularly in healthcare. This study proposes the application of a suitable location model for field hospitals during the novel coronavirus 2019 (COVID-19) pandemic. The used model is the most appropriate among the threemost common locationmodels utilized to solve healthcare problems (the set covering model, the maximal covering model, and the P-median model). The proposed nonlinear binary constrained model is a slight modification of the maximal covering model with a set of nonlinear constraints. The model is used to
Pagination
- Previous page ‹‹
- Page 11
- Next page ››
